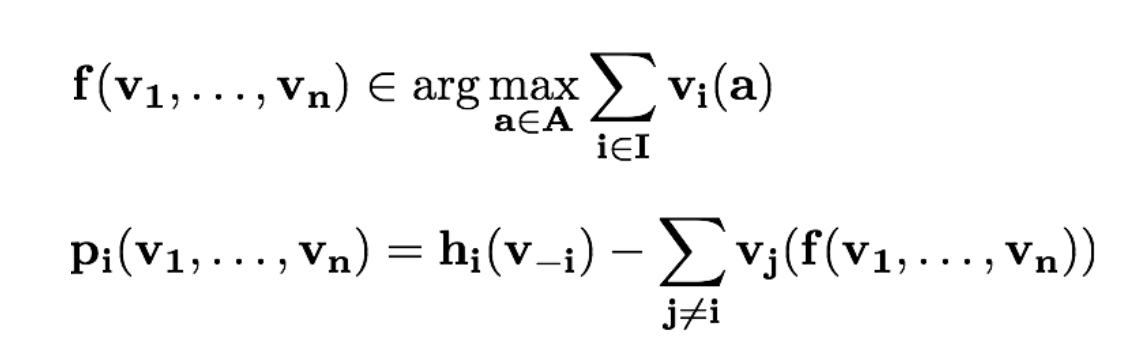Jeremy

Mechanisms with Money

Definition

  1. Finite set of alternatives A={a,b,c,…} Set I of n players

  2. The preference is expressed by a valuation function

  • vi = A -> R

  • vi(a): the “value” in terms of some currency that player i gives to alternative a

  • utility: ui := vi(a) + m

Single Item Auction （拍卖）

  • Goal: Give the object to the player with maximum value.

  • in a way that cannot be strategically manipulated

Description of the Game

  • Strategy Set: The players bid bi (probably ≠ vi)

  • Allocation Algorithm: Who is going to get the object?

    • xi = xi(b) ({0, 1}) // xi(b) 属于 0 或 1

  • Payment Scheme: At what price?

    • p = p(b)

  • player i wants to maximize his utility ui=xi(vi-p)

  • No Payment

    1. p = 0€

    2. b2 > 100€

  • First Price Auction

    • p=100 €

    • p=70+ε €

    • ==> b1 > 70+ε €

Vickrey Auction

  • definition:

    • Allocation Algorithm: Give the object to the player with the highest bid.

    • Payment Scheme: The winner pays an amount equal to the second highest bid.

    Proposition (Vickrey 1961) For every v1 ,…, vn and every v’i, let ui be the utility if he bids vi and u’i his utility if he bids v’i. Then, ui ≥ u’i. (for the utilities we assume vi as the true valuation)

  • Goal: The guy with the highest valuation will get the object!

  • Telling the truth is a dominant strategy for the players.

  • Remark:

    • “This very simple and elegant idea achieves something quite remarkable. It reliably computes a function (argmax) on n numbers (the vi’s) that are each held secretly by a different self-interested player”

Incentive Compatible Mechanisms

  • choose alternative + charge payment

  • vi: A -> R for vi belong to Vi

  • set of valuations for player i Vi is a truth subset of R_A (R_A 是 Vi 的真子集)

  • A (direct revelation) mechanism is a social choice function f:V1×⋅⋅⋅×Vn→A and a vector of payment functions p1,…,pn, where pi:V1×⋅⋅⋅×Vn→R

  • A mechanism (f, p1,…,pn) is incentive compatible (or strategy proof) if for every player i, every v=(v1,…,vn) and every v’i vi(f(vi,v-i))-pi(vi,v-i) ≥ vi(f(v’i,v-i))-pi(v’i,v-i)

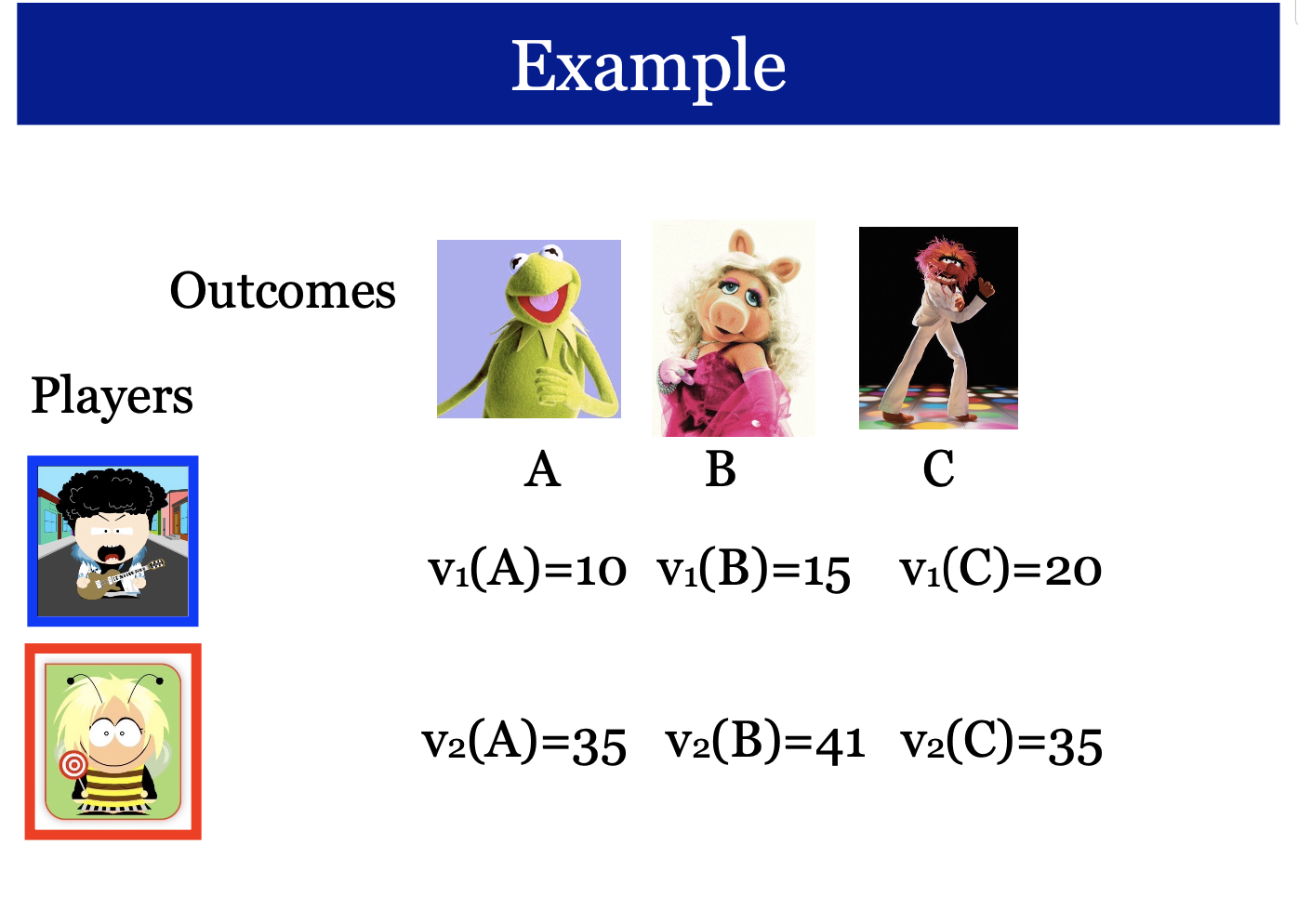
  • Example1:
f(vi,v-i)=a f(v’i,v-i)=b
pi(vi,v-i)=3 pi(v’i,v-i)=5
vi(f(vi,v-i))=6 vi(f(vi,v-i))=7
  • Example2:
f(vi,v-i)=a f(v’i,v-i)=b
pi(vi,v-i)=3 pi(v’i,v-i)=5
vi(f(vi,v-i))=vi(a)=6 vi(f(v’i,v-i))=vi(b)=7
vi(f(vi,v-i))-p(vi,v-i) =3 vi(f(v’i,v-i))-p(v’i,v-i)=2
  • Example3:
f(vi,v-i)=a f(v’i,v-i)=b
pi(vi,v-i)=3 pi(v’i,v-i)=3
vi(f(vi,v-i))=vi(a)=6 vi(f(v’i,v-i))=vi(b)=7
vi(f(vi,v-i))-p(vi,v-i)=3 vi(f(v’i,v-i))-p(v’i,v-i)=4

Vickrey-Clarke-Groves (VCG) Mechanisms

A mechanism (f,p1,…,pn) is called VCG mechanism if

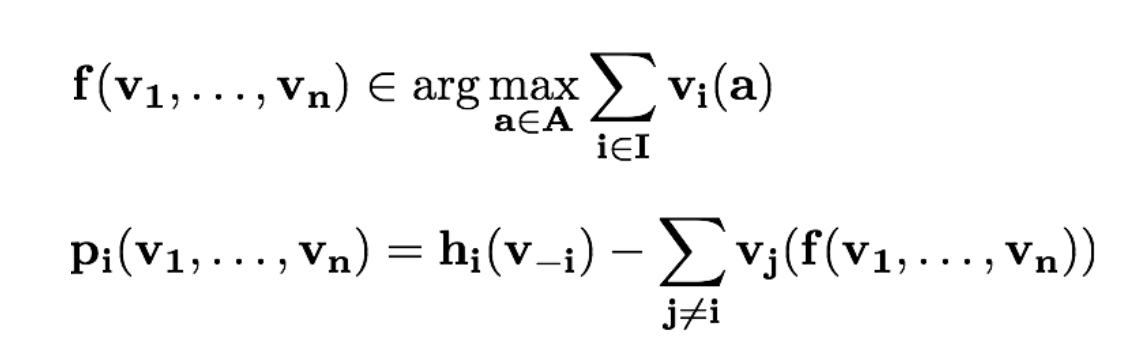
VCG Payments

  • A mechanism is ex-post individually rational if players get non-negative utility.

vi(f(vi,v-i))-pi(vi,v-i) ≥ 0

  • A mechanism has no positive transfers if no player is ever paid money.

pi(vi,v-i) ≥ 0